A quadratic distance bound on sliding between crossing-free spanning trees

O. Aichholzer and K. Reinhardt

Abstract:

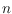
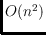
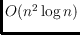
Let $S$ be a set of $n$ points in the plane and let ${\mathcal T}_S$ be the set of all crossing-free spanning trees of $S$. We show that any two trees in ${\mathcal T}_S$ can be transformed into each other by $O(n^2)$ local and constant-size edge slide operations. No polynomial upper bound for this task has been known, but in [#!AAH!#] a bound of $O(n^2 \log n)$ operations was conjectured.

Reference: O. Aichholzer and K. Reinhardt. A quadratic distance bound on sliding between crossing-free spanning trees. Computational Geometry: Theory and Applications, special issue, 37:155-161, 2007.