Generalized self-approaching curves

O. Aichholzer, F. Aurenhammer, C. Icking, R. Klein, E. Langetepe, and G. Rote

Abstract:

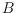
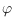
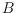
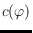
We consider all planar oriented curves that have the following property. For each point $B$ on the curve, the rest of the curve lies inside a wedge of angle $\varphi$ with apex in $B$, where $\varphi < \Pi$ is fixed. This property restrains the curve's meandering. we provide an upper bound $c(\varphi)$ for the length of such a curve, divided by the distance between its endpoints, and prove this bound to be tight. 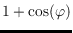A main step is in proving that the curve's length cannot exceed the perimeter of its convex hull, divided by $1+\cos(\varphi)$.

Reference: O. Aichholzer, F. Aurenhammer, C. Icking, R. Klein, E. Langetepe, and G. Rote. Generalized self-approaching curves. In Proc. $14^{th}$ European Workshop on Computational Geometry CG '98, pages 15-18, Barcelona, Spain, 1998.