O. Aichholzer, D. Alberts, F. Aurenhammer, and B. Gärtner
A new internal structure for simple polygons, the straight skeleton, is
introduced and discussed. It is composed of pieces of angular bisectores
which partition the interior of a given
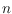
-gon
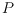
in a tree-like fashion
into
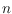
monotone polygons. Its straight-line structure and its lower
combinatorial complexity may make the straight skeleton preferable to the
widely used medial axis of a polygon. As a seemingly unrelated application,
the straight skeleton provides a canonical way of constructing a polygonal
roof above a general layout of ground walls.